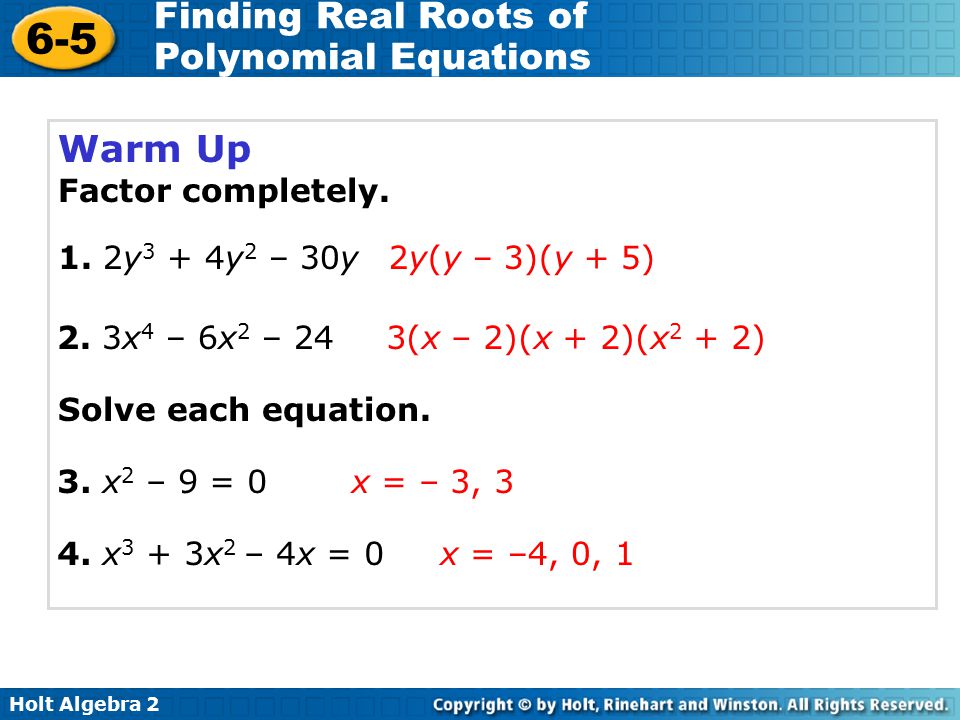
How to Determine How Many Real Roots a Polynomial Has
The highest power ie degree of equation is 3. Given the number of roots you know if there is at least one.
Finding Real Roots Of Polynomial Equations Ppt Download
In this case a 1 b 0 and c -4.
. Just by counting how many times the sign changes from plus to minus or minus to plus. If a and c have the same signs both are positive or both are negative then the quadratic equation has two complex roots. The discriminant of a quadratic ax2bxc is given by the formula.
The signs of the coefficients are in the pattern. X3-2x210x1360 2 Use the rational root theorem to list all problem rational roots of the polynomial equation. 2x3 x2 7x 2 x 2 2x 2 3x 1 Now we can get the roots of the above polynomial since we have got one linear equation and one quadratic equation for which we know the formula.
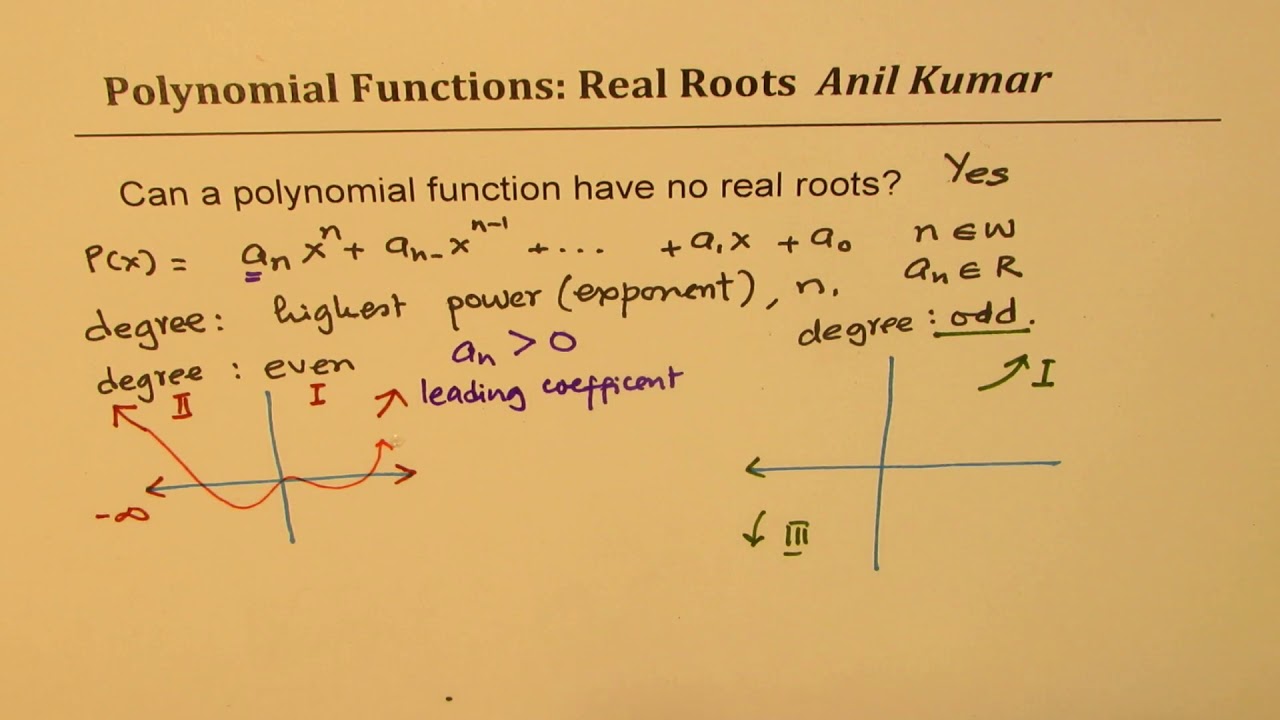
Find roots of any function step-by-step. If none of the roots is 0 ie if a_0 neq 0 then each root is at least 1 in absolute value and thus a_0 is an upper bound on every product of the form prod_i in S r_i. To determine the possible number of negative Real zeros look at the signs of the coefficients of f x.
Consider the cubic equation where a b c and d are real coefficients. Given function is arranged in descending powers of the variable. 2 23 4 22 3 2 6 2 8 4 4 6 6 0.
Now the equation is. A polynomial of degree 1 has 1 root. Now we have to divide polynomial with x ROOT.
Get step-by-step solutions from expert tutors as fast as 15-30 minutes. Therefore the total number of roots when counting. When a real root has.
Namely if fx factors as fx prod_i x r_i then we know a_0 prod_i r_i. Your first 5 questions are on us. Divide the given polynomial by x 2 since it is one of the factors.
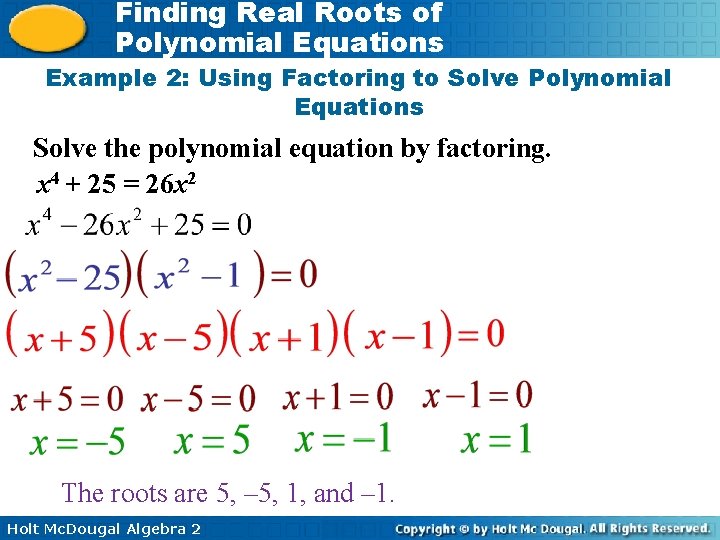
Finding Real Roots of Polynomial Equations Sometimes a polynomial equation has a factor that appears more than once. According to Descartes rule of signs. Number of Roots of a Polynomial.
2x3 x2 7x 2 x 2 2x2 3x 1 Now that we have one linear equation and one quadratic equation for which we know the formula we can find the roots of the aforementioned polynomial. The complex polynomial x2 1 has two roots. However we can only count two real roots.
For example in the equation we have a polynomial of degree four. Do not find the actual roots. 2x3 4x2 3x 6 x 2 2x2 3.
Sturms theorem lets you calculate the number of real roots in the range a b. So the concept of root for a polynomial depends not just on the polynomial but also on the field in question. I three distinct real roots ii one pair of repeated roots and a distinct root iii one real root and a pair of conjugate complex roots.
The part counted with multiplicity means that we have to count the roots by their multiplicity that is by the times they are repeated. If the discriminant is negative the polynomial has two complexroots and of course no real roots. This is because the root at is a multiple root with a multiplicity of three.
For example the root 0 is a factor three times because 3x3 0. Hence number of roots is the highest power in the equation. Σa b aΣαβ c a Σ a b a Σ α β c a.
Delta b2-4ac Then. From the bottom half of page 4 of this paper. 3 - 5 5 5 2.
This equation has either. Denote it by f0x and its derivative fx by f1x. F x x4 x3 x2 x 2.
Has roots αβγ α β γ then. The real polynomial x2 1 has no roots. It is isomorphic to R n.
2x2 3 2x2 x2 x1. The degree of a polynomial is the highest power of its monomials with non-zero coefficients. Every polynomial has a.
Here are the coefficients of our variable in fx. For example the quadratic equation x 2 4 0 has two real roots. How many roots does the polynomial 3 𝑥 1 𝑥 4 𝑥 2 have.
Using the fundamental theorem of algebra the number of roots is equal to the degree of the polynomial. So x 2 is the root of the equation. The multiplicity of root r is the number of times that x r is a factor of Px.
Ths discriminant gives less information for polynomials of higher degree. Also every time you cross Δ the discriminant switches signs. In this case we have been given a polynomial in factored form.
This is the same as reversing the sign on terms of odd degree. If you want to know how many real roots a polynomial with real coefficients has then you might like to look at the discriminant - especially if the polynomial is a quadratic or cubic. If the discriminant is zero the polynomial has one doublereal root.
Determine the number of positive and negative real zeros for the given function. There is a hypersurface Δ in P cut out by the equation of the discriminant. As the number of roots for an equation is equal to degree.
This creates a multiple root. Now we use 2x2 - 3 to find remaining roots. Let P be the space of all real monic polynomials of degree n.
Every polynomial of degree n except for 0 has exactly n roots if and only if the field in question is algebraically closed. In 3x5 18x4 27x3 0 has two multiple roots 0 and 3. It is used to determine the number of real zeros of a polynomial function.
Hence there are 3 roots of the given equation. Ax3 bx2 cxd 0 a x 3 b x 2 c x d 0. Roots of cubic polynomials.
Since there are 3 changes of sign there are 3 or 1. Let fx be a real polynomial. 1 Find the roots of the polynomial equation.
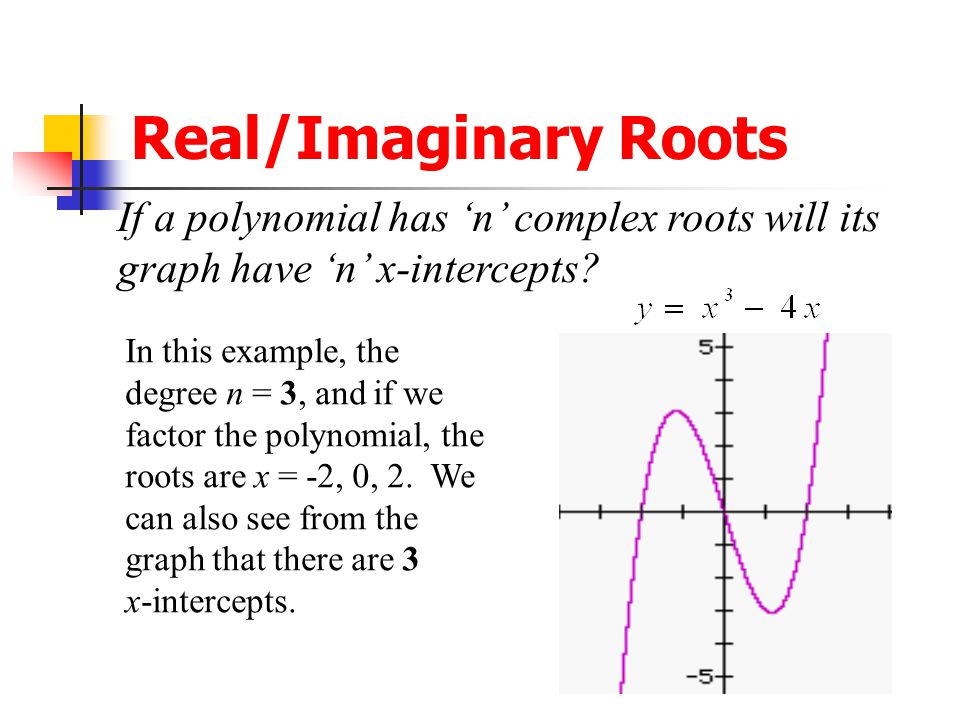
Proceed as in Euclids algorithm to find. As you cross Δ the number of real roots goes up or down by 2. Polynomials have roots zeros where they are equal to 0.
If a and c have opposite signs one is positive and the other is negative then the quadratic equation has real roots. In this case we divide 2x3 x2 3x 6 by x 2. If you mean a square trinomialthenIf the discriminant is positive the polynomial has two realroots.
Roots are at x2 and x4 It has 2 roots and both are positive 2 and 4 Sometimes we may not know where the roots are but we can say how many are positive or negative.

6 5 6 6 Theorems About Roots And The Fundamental Theorem Of Algebra Ppt Download
Finding Real Roots Of Polynomial Equations How Do

Finding Zeros Of Polynomials 1 Of 2 Video Khan Academy

Find The Roots Of The Following Polynomial Equations Given One Of The Roots On The Right Side Youtube
Roots Zeros Of Polynomials Ppt Video Online Download

Real Roots Of Polynomial Functions Youtube
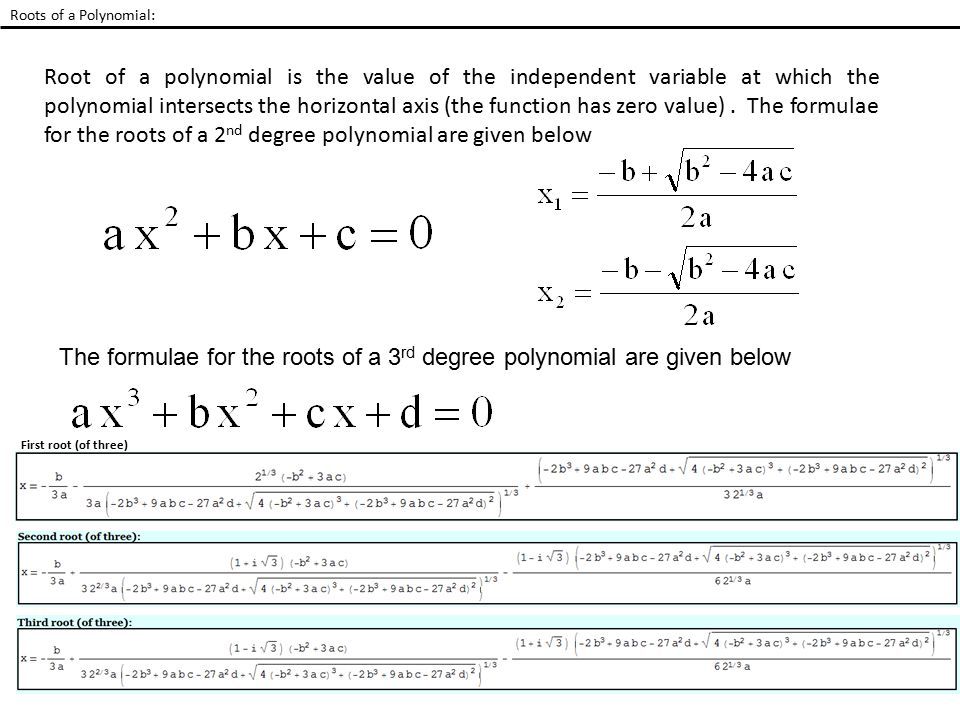
Roots Of A Polynomial Root Of A Polynomial Is The Value Of The Independent Variable At Which The Polynomial Intersects The Horizontal Axis The Function Ppt Telecharger

How To Solve Higher Degree Polynomials With Pictures Wikihow

Finding Square Root Of A Polynomial

Real Zeros Of Polynomial Functions Ppt Video Online Download
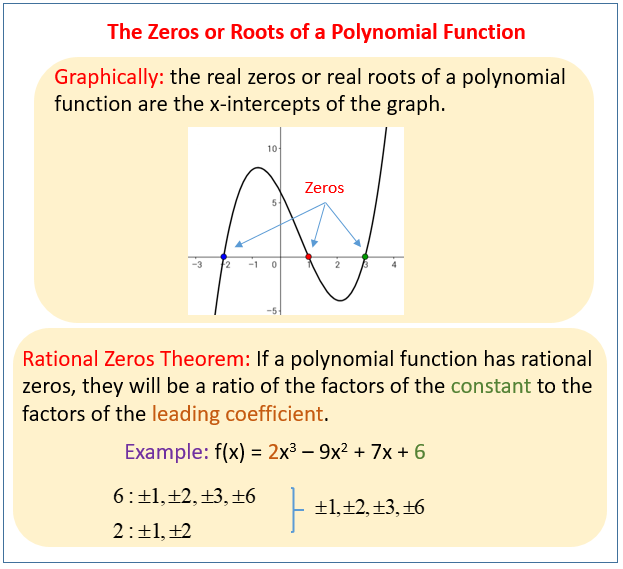
Find Zeros Of A Polynomial Function Solutions Examples Videos Worksheets Solutions Activities

Complex Roots Of Polynomials Youtube
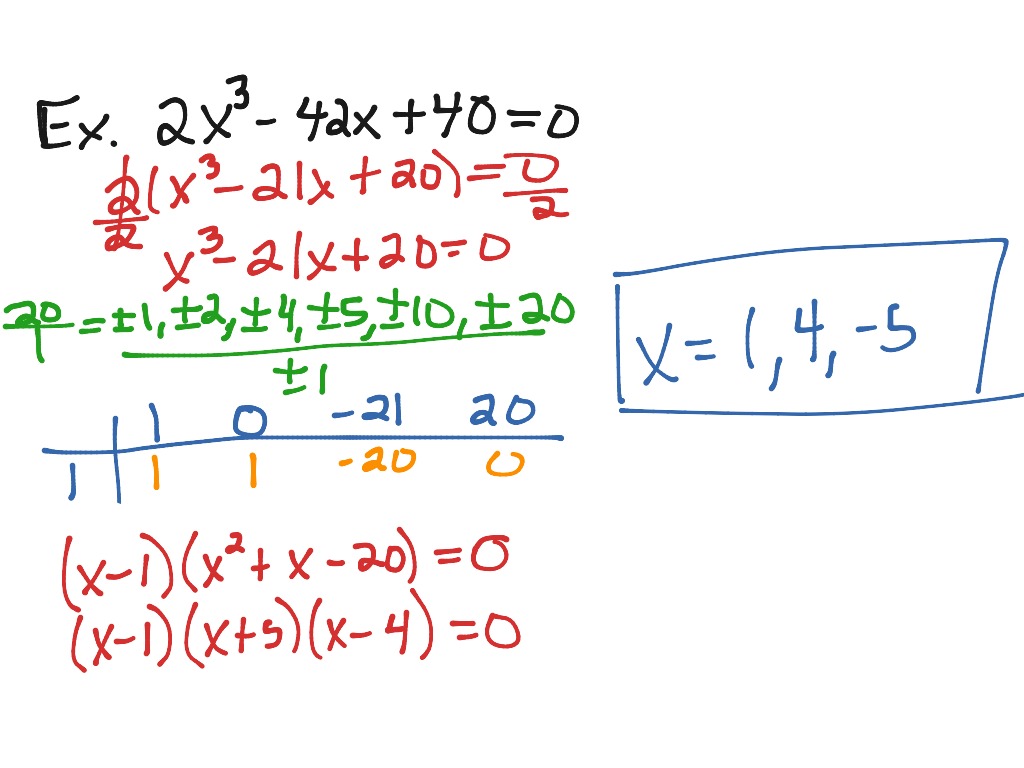
Finding Real Roots Of Polynomials Math Algebra 2 Finding Real Roots Of Polynomial Equations Rational Root Theorem Showme

Roots Zeros Of Polynomials Ii Finding The Rootszeros

Can Polynomial Functions Have No Real Roots Youtube
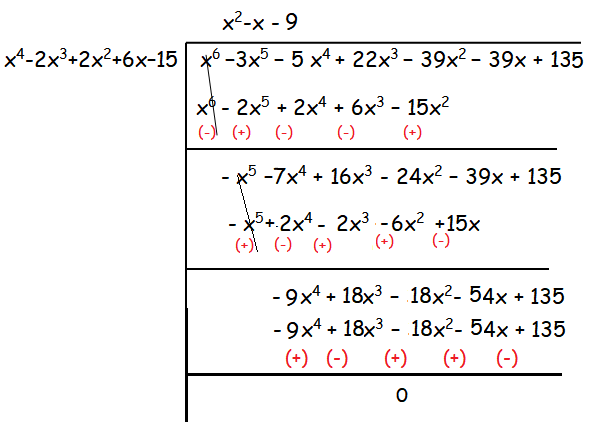
Find The Other Roots Of The Polynomial Equation Of Degree 6

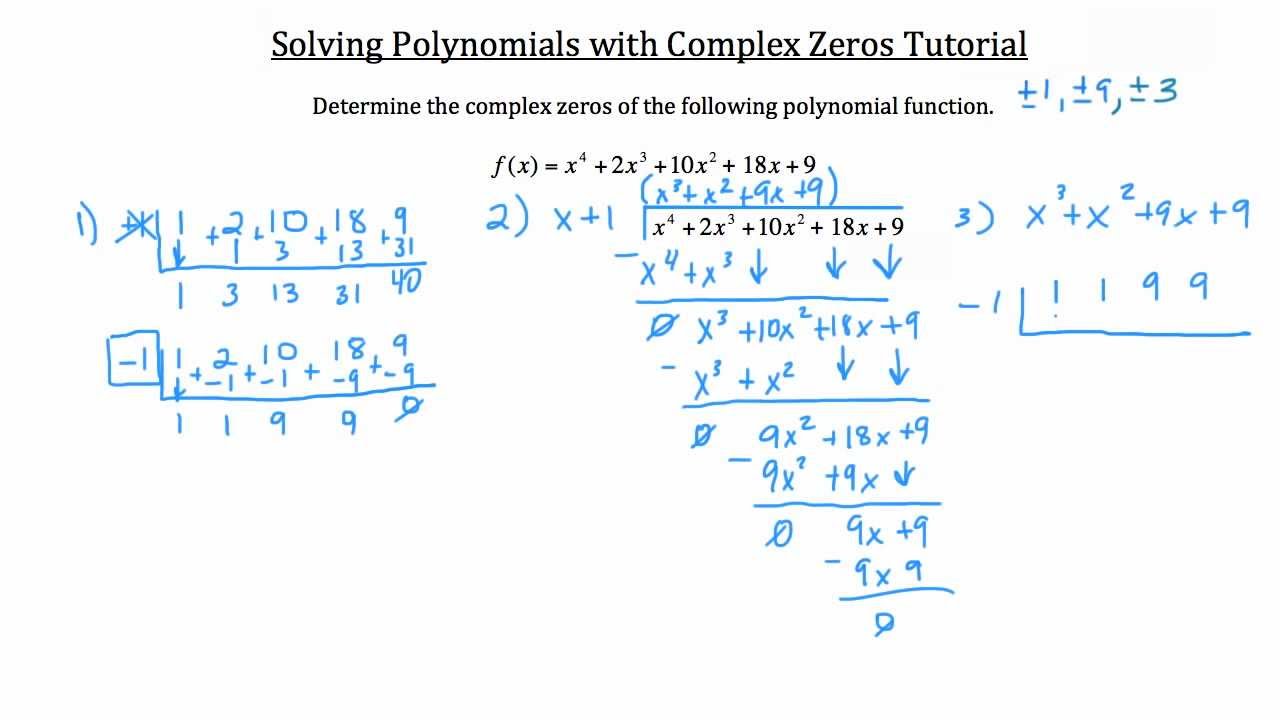
Comments
Post a Comment